Speaker
Description
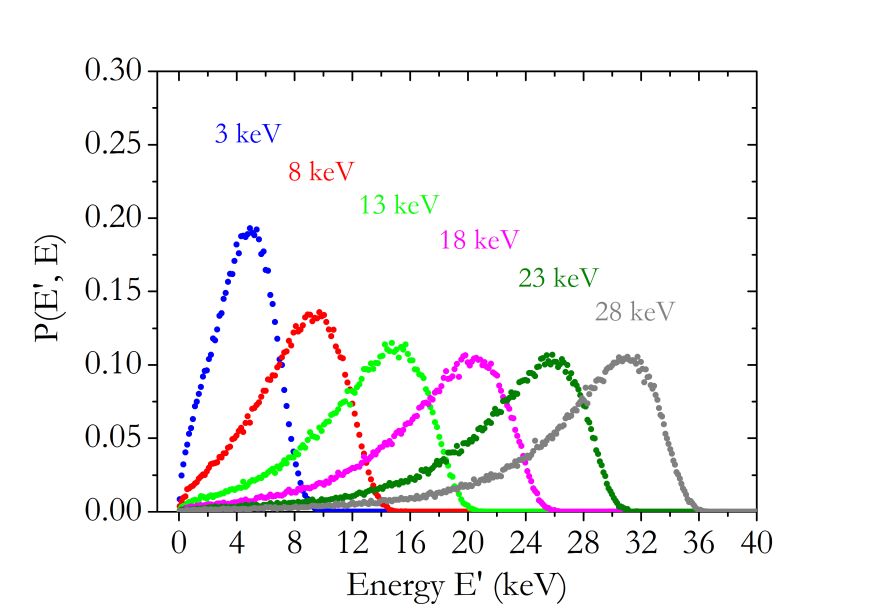
The analysis of depth-dependent data of thin-film film semiconductor heterostructures is discussed in this work. The data is obtained by varying muon implantation energy, E, using the Low Energy Muon facility (LEM) at PSI, Switzerland. Since the measurement method has a finite resolution, deconvolution of the measured profile with the resolution function is required. The unfolding can be implemented in the real space (that is in depth variable x), using range distribution function, P(x,E), obtained by Monte Carmo simulations. The unfolding in depth space requires detailed knowledge of the sample which may not be available. It is shown that it is much simpler to start by performing the deconvolution in the implantation energy space and to transform it afterwards into real space. This requires transforming P(x,E), in P(E’,E) by an adequate variable change x = f(E'). The best approach is to use the relation between the median and the implantation energy for the coordinate transformation, since it ensures that the range distribution is centered at the implantation energy E. The range distributions in energy space, as derived from the depth distributions in CdS, are depictured in the Fig.1. They are considered nearly universal for all materials.